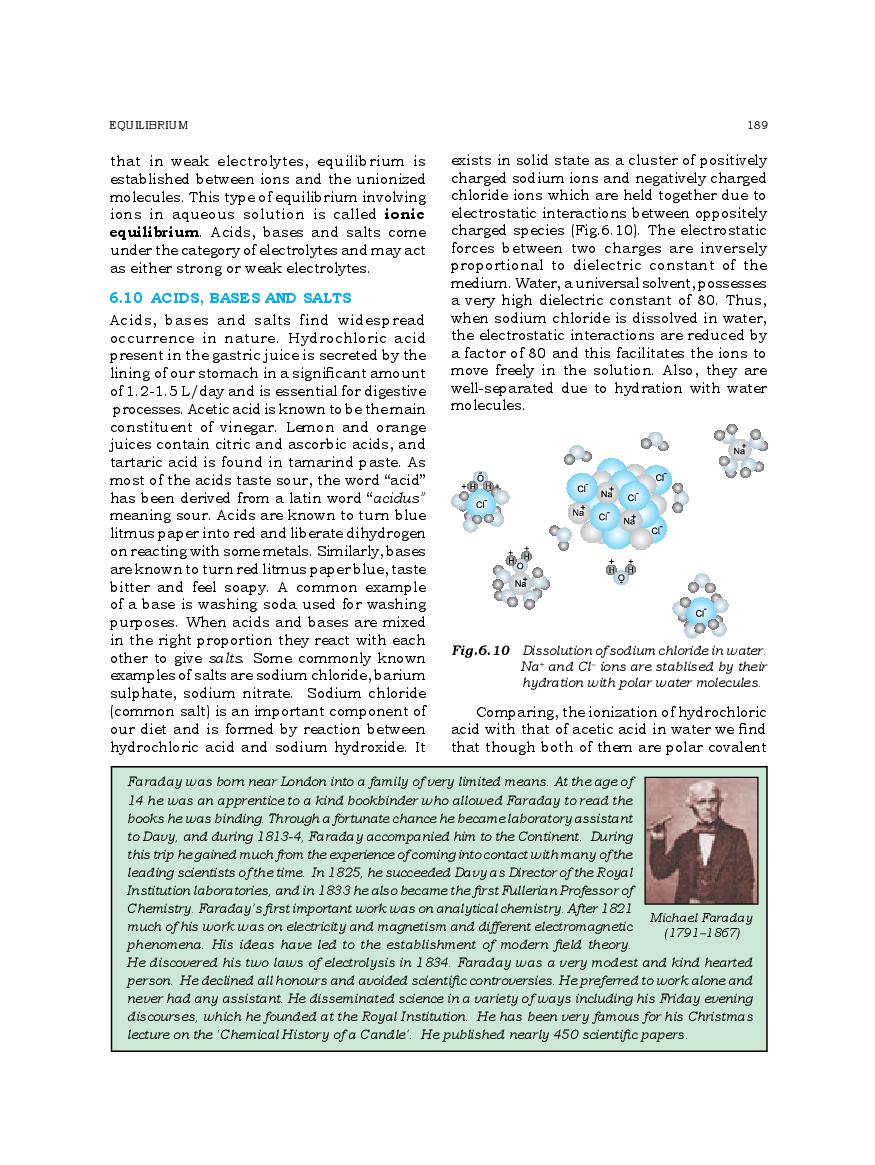
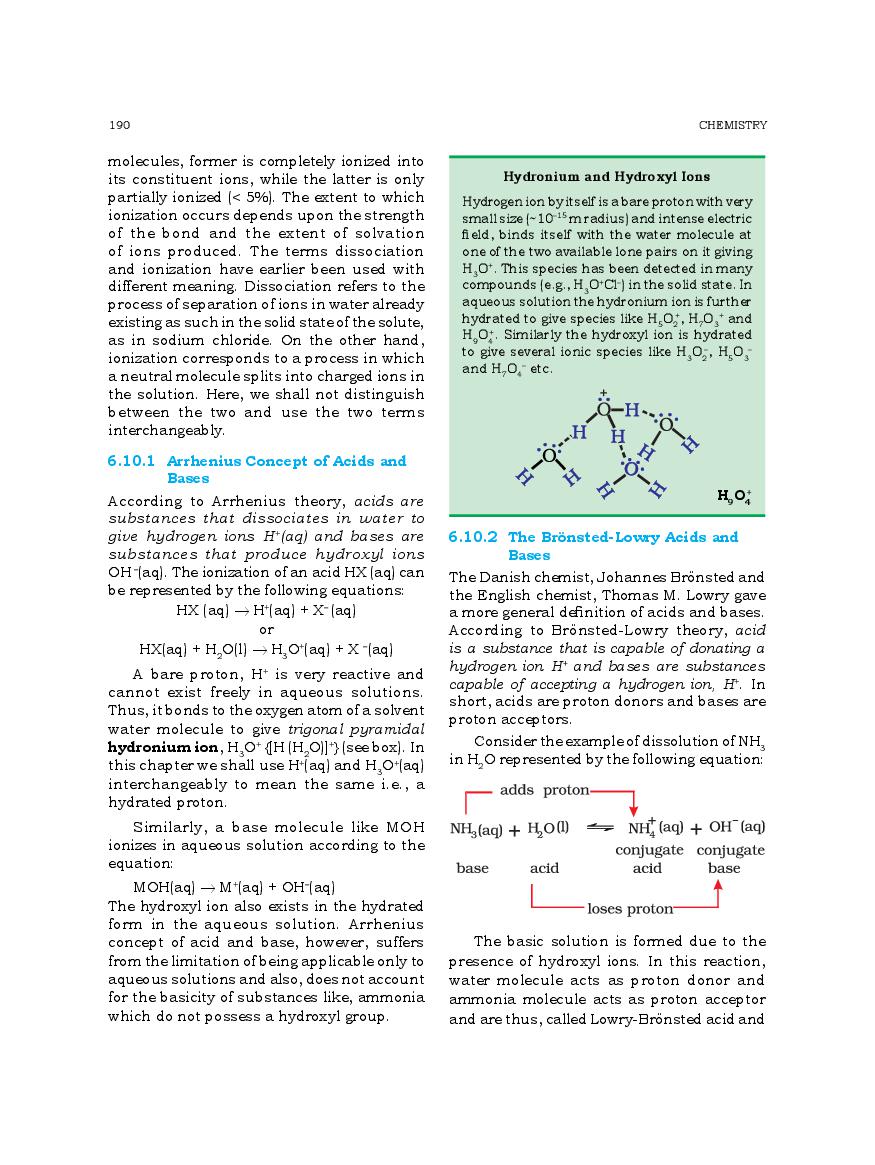
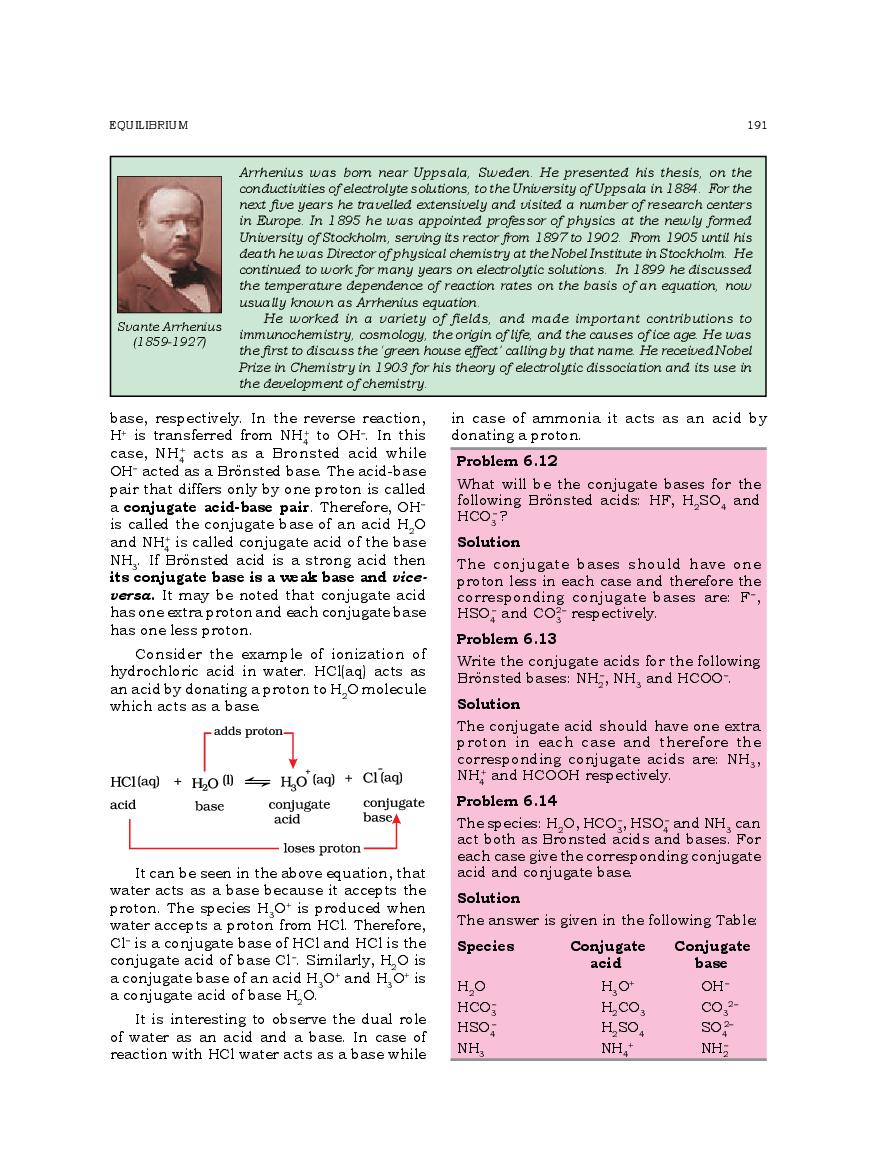
NCERT Book Class 11 Chemistry Chapter 6 Equilibrium is here. You can read and download Class 11 Chemistry Chapter 6 PDF from this page of aglasem.com. Equilibrium is one of the many lessons in NCERT Book Class 11 Chemistry in the new, updated version of 2023-24. So if you are in 11th standard, and studying Chemistry textbook (named Chemistry), then you can read Ch 6 here and afterwards use NCERT Solutions to solve questions answers of Equilibrium.
NCERT Book Class 11 Chemistry Chapter 6 Equilibrium
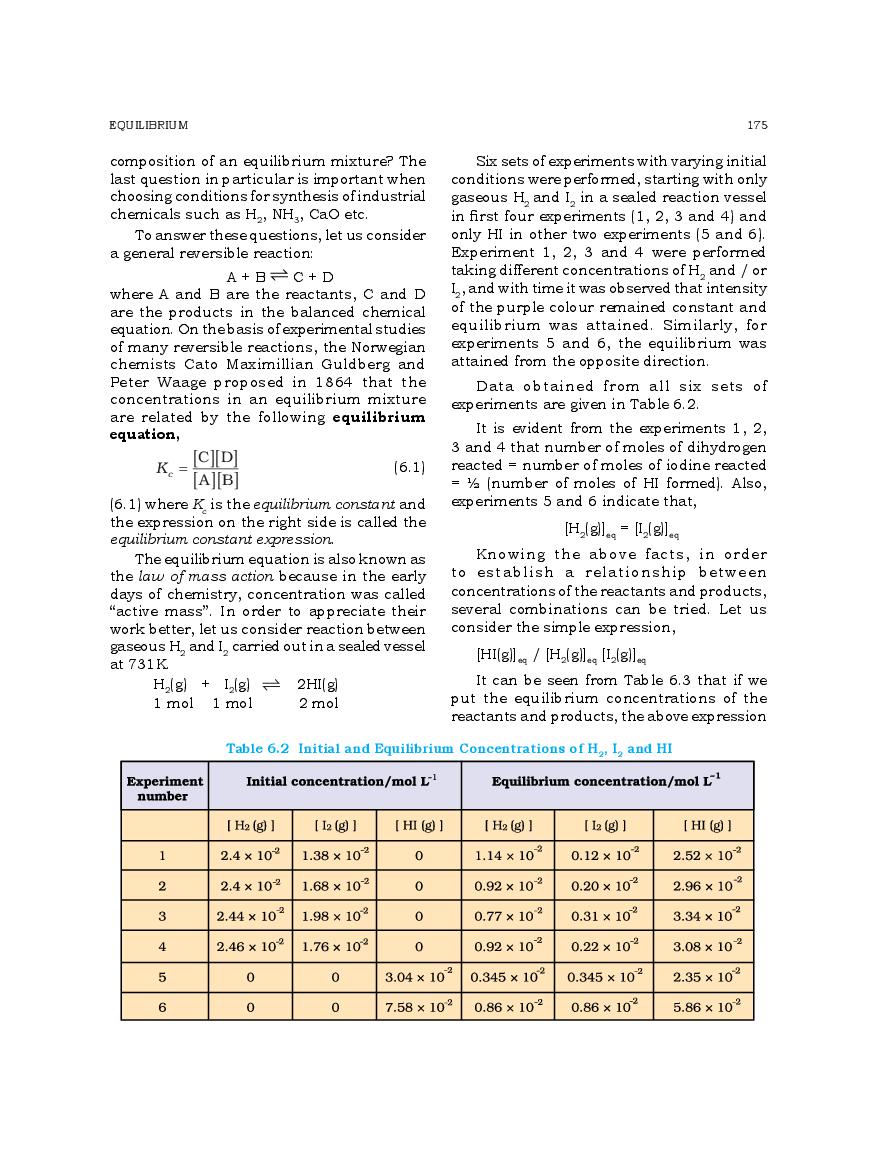
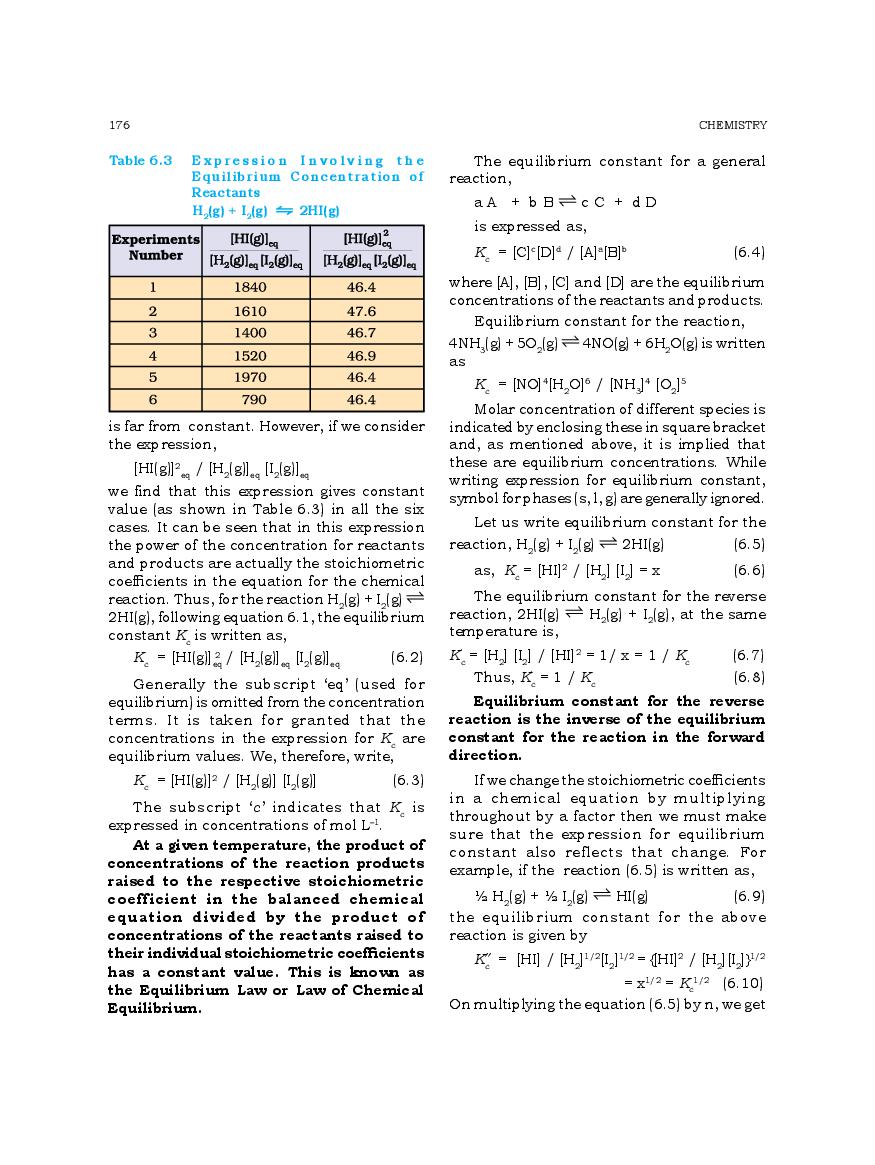
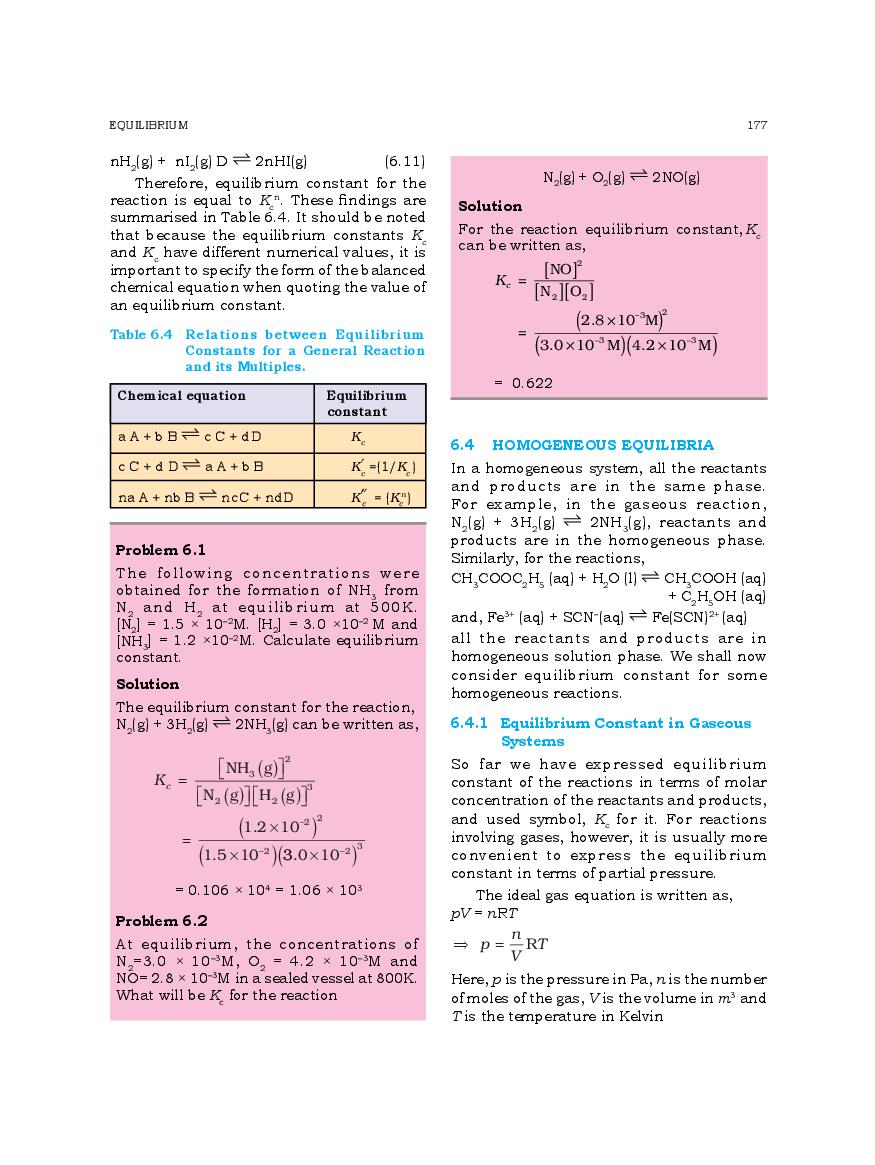
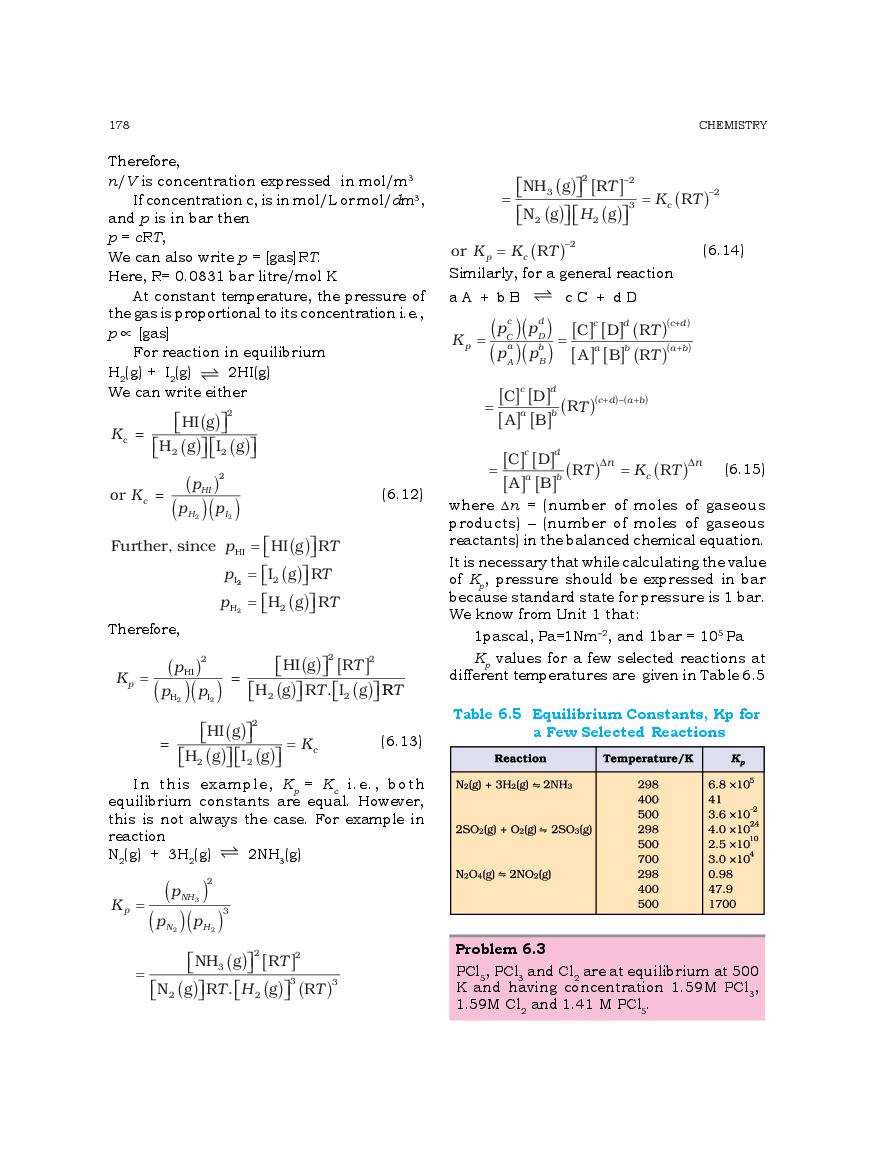
The complete Chapter 6, which is Equilibrium, from NCERT Books for Class 11 Chemistry is as follows.
NCERT Book Class 11 Chemistry Chapter 6 Equilibrium PDF Download Link – Click Here To Download The Complete Chapter PDF
NCERT Book Class 11 Chemistry Full Book PDF Download Link – Click Here To Download The Complete Book PDF
NCERT Book Class 11 Chemistry Chapter 6 Equilibrium PDF
The direct link to download class 11 Chemistry NCERT Book PDF for chapter 6 Equilibrium is given above. However if you want to read the complete lesson on Equilibrium then that is also possible here at aglasem. So here is the complete class 11 Chemistry Ch 6 Equilibrium.
NCERT Book Class 11 Chemistry Chapter 6 Equilibrium View DownloadNCERT Book for Class 11 Chemistry
Besides the chapter on Equilibrium, you can read or download the NCERT Class 11 Chemistry PDF full book from aglasem. Here is the complete book:
- Chapter 1: Some Basic Concepts Of Chemistry
- Chapter 2: Structure Of Atom
- Chapter 3: Classification of Elements and Periodicity
- Chapter 4: Chemical Bonding And Molecular Structure
- Chapter 5: Thermodynamics
- Chapter 6: Equilibrium
- Chapter 7: Redox Reactions
- Chapter 8: Organic Chemistry- Some Basic Principles And Techniques
- Chapter 9: Hydrocarbons
NCERT Books for Class 11
Similarly all the subject-wise class 11 books at aglasem.com are as follows.
- NCERT Book Class 11 Accountancy
- NCERT Book Class 11 Biology
- NCERT Book Class 11 Business Studies
- NCERT Book Class 11 Chemistry
- NCERT Book Class 11 Economics
- NCERT Book Class 11 English
- NCERT Book Class 11 Geography
- NCERT Book Class 11 Hindi
- NCERT Book Class 11 History
- NCERT Book Class 11 Maths
- NCERT Book Class 11 Physics
- NCERT Book Class 11 Political Science
- NCERT Book Class 11 Psychology
- NCERT Book Class 11 Sociology
NCERT Books
All class-wise books of National Council of Educational Research and Training are as follows.
- NCERT Books for Class 1
- NCERT Books for Class 2
- NCERT Books for Class 3
- NCERT Books for Class 4
- NCERT Books for Class 5
- NCERT Books for Class 6
- NCERT Books for Class 7
- NCERT Books for Class 8
- NCERT Books for Class 9
- NCERT Books for Class 10
- NCERT Books for Class 11
- NCERT Books for Class 12
Class 11 Chemistry Chapter 6 Equilibrium NCERT Textbook – An Overview
The highlights of this Equilibrium chapter PDF are as follows.
| Aspects | Details |
|---|---|
| Class | 11 |
| Subject | Chemistry |
| Book | Chemistry |
| Chapter Number | Ch 6 |
| Chapter Name | Equilibrium |
| Book Portion Here | NCERT Book Class 11 Chemistry Ch 6 Equilibrium |
| Download Format | |
| Version | NCERT Book (New, Updated) 2023-24 |
| Complete Book | NCERT Book Class 11 Chemistry |
| All Class 11 Books | NCERT Books for Class 11 |
| All Textbooks | NCERT Books |
| NCERT Books in Hindi | NCERT Books for Class 11 in Hindi |
| NCERT Solutions | NCERT Solutions for Class 11 |
| More Study Material | Class 11 |
If you have any queries on NCERT Book Class 11 Chemistry Chapter 6 Equilibrium, then please ask in comments below. And if you found the Class 11 Chemistry Chapter 6 Equilibrium PDF helpful, then do share with your friends on telegram, facebook, whatsapp, twitter, and other social media! :)
To get study material, exam alerts and news, join our Whatsapp Channel.