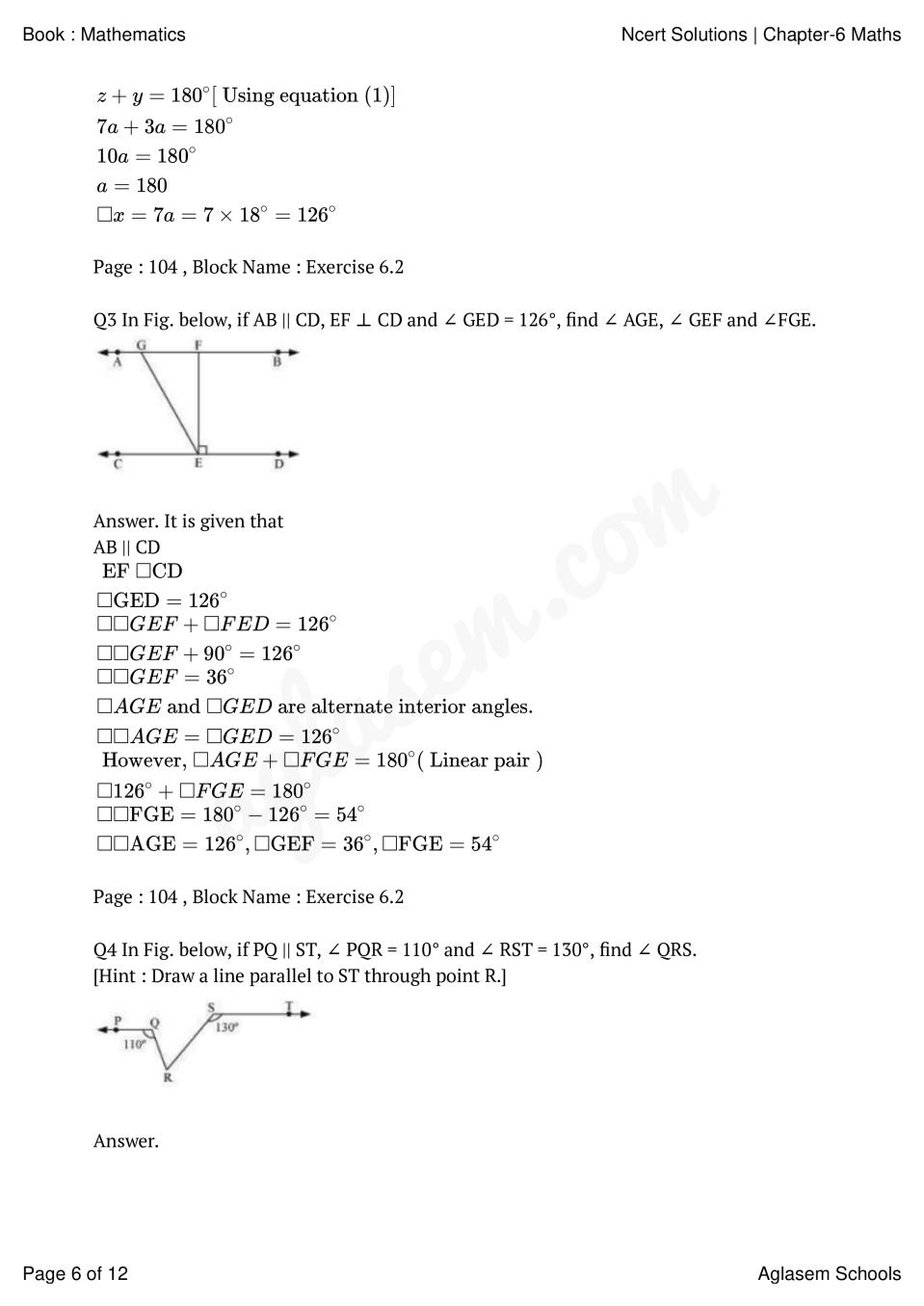
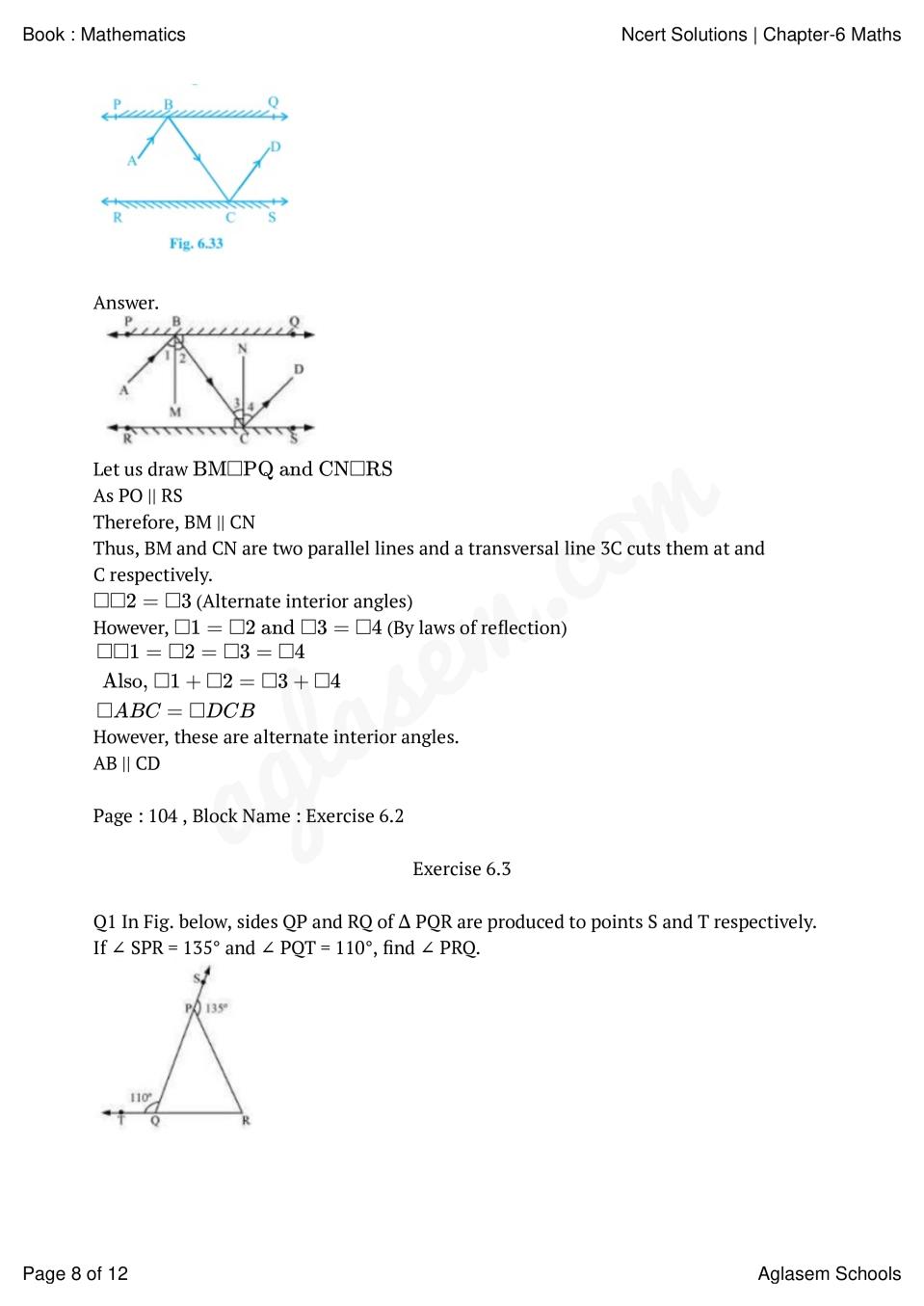
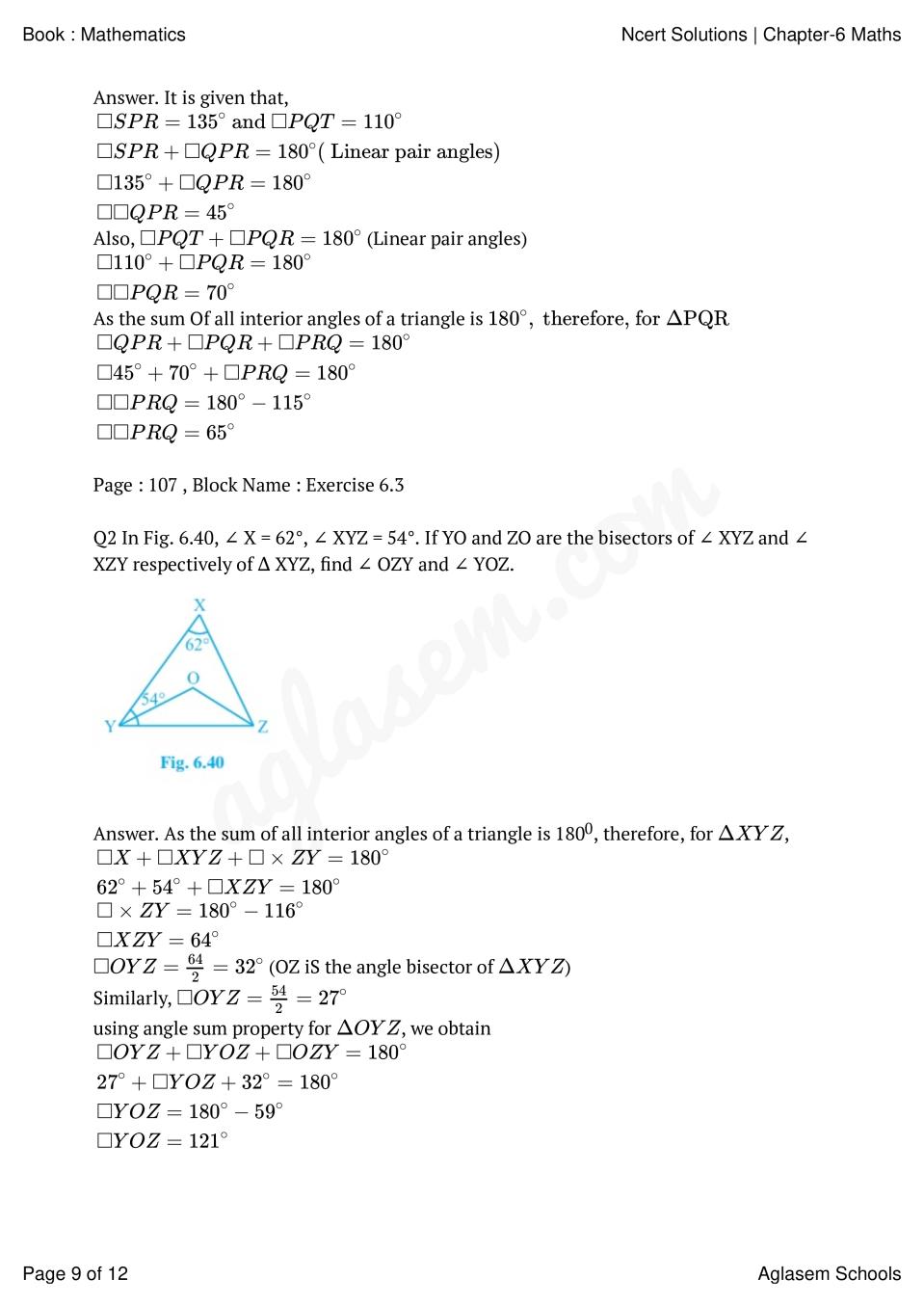
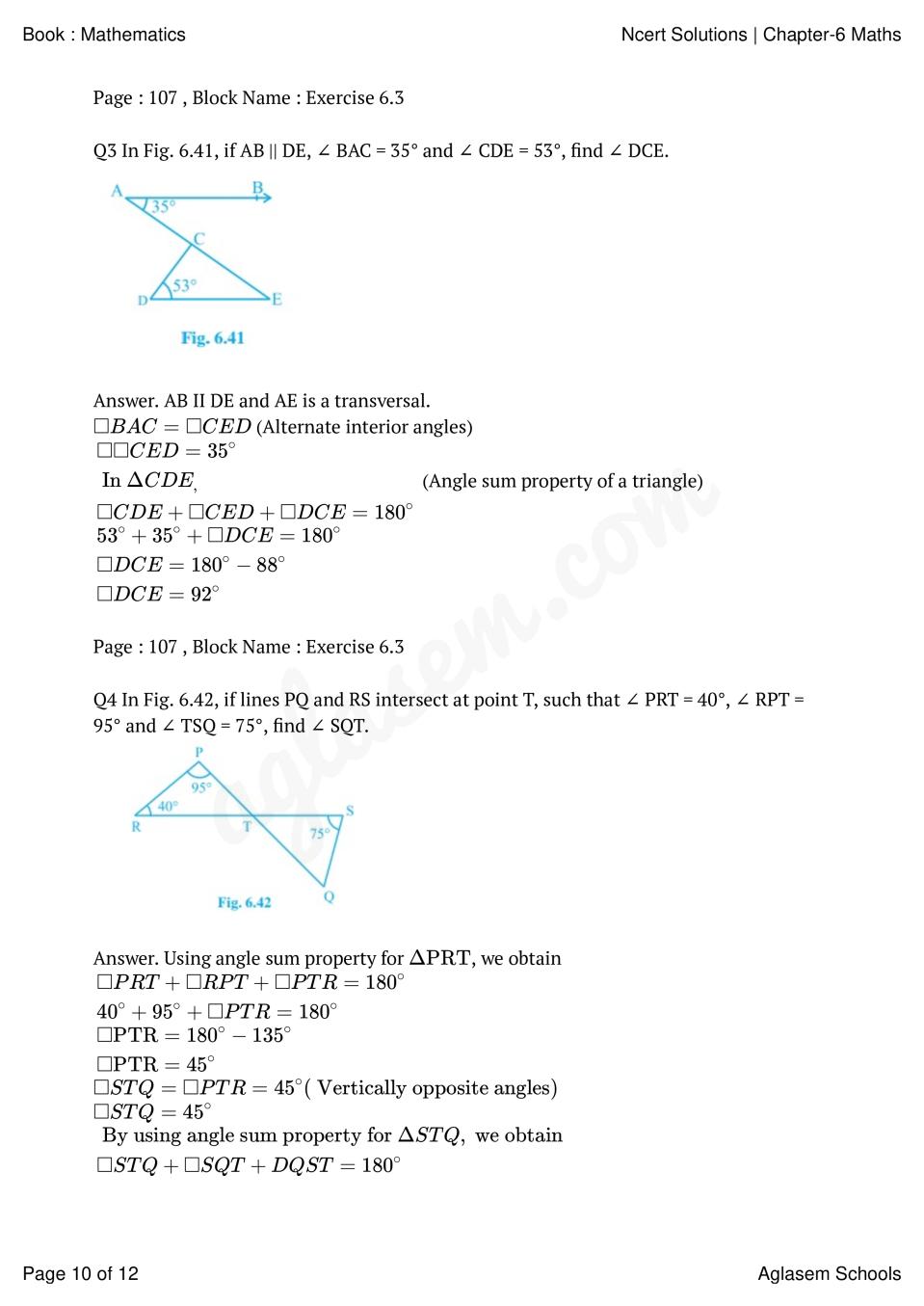
NCERT Solutions for Class 9 Maths Chapter 6 Lines and Angles has been published by Aglasem. You can now download the Class 9 Maths Ch 6 Questions and Answers PDF here. This NCERT Solutions for Class 9 Maths contains answers of all questions asked in Chapter 6 in textbook, Mathematics. Therefore you can refer it to solve Lines and Angles exercise questions and learn more about the topic.
NCERT Solutions for Class 9 Maths Chapter 6 Lines and Angles
Class – Class 9
Subject – Maths
Chapter – Ch 6
Chapter Name – Lines and Angles
Book – Mathematics
Study Material – NCERT Solutions
NCERT Solutions for Class 9 Maths Chapter 6 PDF
While you can read NCERT Solutions for Class 9 Maths Ch 6 for all exercises here on aglasem. You can also download this NCERT Solutions PDF to refer at anytime when you study Lines and Angles. Here it is.
NCERT Solutions for Class 9 Maths Chapter 6 PDF Download Link – Click Here to Download Solutions PDF
How to download NCERT Solutions for Class 9 Maths Chapter 6 PDF?
You can download the complete NCERT solutions for chapter 6 of this NCERT Book i.e. Mathematics with following steps.
- First search NCERT Solutions for Class 9 Maths Ch 6 PDF aglasem and come to this page.
- Now you will see the exercise questions answers of Lines and Angles and download pdf link on it.
- Click the Download PDF link to obtain the Lines and Angles questions with answers document.
NCERT Solutions for Class 9 Maths
There are more chapters to study besides Lines and Angles in this subject. So here are NCERT solutions for all topics of Maths taught in 9th class here at aglasem.
- Chapter 1 Number Systems
- Chapter 2 Polynomials
- Chapter 3 Coordinate Geometry
- Chapter 4 Linear Equation in Two Variables
- Chapter 5 Introduction to Euclid’s Geometry
- Chapter 6 Lines and Angles
- Chapter 7 Triangles
- Chapter 8 Quadrilaterals
- Chapter 9 Circles
- Chapter 10 Heron’s Formula
- Chapter 11 Surface Area and Volume
- Chapter 12 Statistics
NCERT Solutions for Class 9
Just like you got Maths Ch 6 solutions here. You can see exercise questions answers of other subjects and their topics too on aglasem. Here are NCERT solutions for all subjects of 9th standard NCERT books.
NCERT Solutions for Class 9 Maths Chapter 6 – An Overview
The key highlights of this study material are as follows.
| Aspects | Details |
|---|---|
| Class | Class 9 |
| Subject | Maths |
| Chapter Number | Ch 6 |
| Chapter Name | Lines and Angles |
| Book Name | Mathematics |
| Book By | NCERT (National Council of Educational Research and Training) |
| Educational Resource Here | NCERT Solutions of Class 9 Maths Ch 6 for All Exercise |
| More Questions Answers of This Subject | NCERT Solutions for Class 9 Maths |
| Download Book Chapter PDF | NCERT Book Class 9 Maths Chapter 6 |
| All Questions Answers For This Class | NCERT Solutions for Class 9 |
| Complete Solutions | NCERT Solutions |
If you have any queries on NCERT Solutions for Class 9 Maths Chapter 6 Lines and Angles, then please ask in comments below.
To get study material, exam alerts and news, join our Whatsapp Channel.