NCERT Exemplar Class 11 Maths Unit 11 Conic Sections

Candidates can download NCERT Exemplar Class 11 Maths Unit 11 from this page. The exemplar has been provided by the National Council of Educational Research & Training (NCERT) and the candidates can check it from below for free of cost. It contains objective, very short answer type, short answer type, and long answer type questions. Along with it, the answer for each question has also been provided. From the NCERT Exemplar Class 11 Maths Unit 11, candidates can understand the level and type of questions that are asked in the exam.

Click Here for Class 11 Maths Notes

NCERT Exemplar Class 11 Maths Unit 11 Conic Sections

NCERT Exemplar Class 11 Maths Unit 11 is for Conic Sections. The type of questions that will be asked from NCERT Class 11 Maths Unit 11 are displayed in the below provided NCERT Exemplar Class 11 Maths Unit 11. With the help of it, candidates can prepare well for the examination.

Also Check: NCERT Solutions for Class 11 Mathematics

1.1 Overview
11.1.1 Sections of a cone Let l be a fixed vertical line and m be another line intersecting it at a fixed point V and inclined to it at an angle α (Fig. 11.1).

Suppose we rotate the line m around the line l in such a way that the angle α remains constant. Then the surface generated is a double-napped right circular hollow cone herein after referred as cone and extending indefinitely in both directions (Fig. 11.2).

The point V is called the vertex; the line l is the axis of the cone. The rotating line m is called a generator of the cone. The vertex separates the cone into two parts called nappes.
If we take the intersection of a plane with a cone, the section so obtained is called a conic section. Thus, conic sections are the curves obtained by intersecting a right circular cone by a plane.

We obtain different kinds of conic sections depending on the position of the intersecting plane with respect to the cone and the angle made by it with the vertical axis of the cone. Let β be the angle made by the intersecting plane with the vertical axis of the cone (Fig.11.3).

The intersection of the plane with the cone can take place either at the vertex of the cone or at any other part of the nappe either below or above the vertex. When the plane cuts the nappe (other than the vertex) of the cone, we have the following situations:
(a) When β = 90 degree , the section is a circle.
(b) When α < β < 90 degree , the section is an ellipse.
(c) When β = α; the section is a parabola. (In each of the above three situations, the plane cuts entirely across one nappe of the cone).
(d) When 0 ≤ β < α; the plane cuts through both the nappes and the curves of intersection is a hyperbola.

Indeed these curves are important tools for present day exploration of outer space and
also for research into the behaviour of atomic particles.
We take conic sections as plane curves. For this purpose, it is convenient to use equivalent definition that refer only to the plane in which the curve lies, and refer to special points and lines in this plane called foci and directrices. According to this approach, parabola, ellipse and hyperbola are defined in terms of a fixed point (called focus) and fixed line (called directrix) in the plane.
If S is the focus and l is the directrix, then the set of all points in the plane whose
distance from S bears a constant ratio e called eccentricity to their distance from l is a
conic section.
As special case of ellipse, we obtain circle for which e = 0 and hence we study it
differently.

11.1.2 Circle A circle is the set of all points in a plane which are at a fixed distance
from a fixed point in the plane. The fixed point is called the centre of the circle and the
distance from centre to any point on the circle is called the radius of the circle.

Note: The general equation of the circle involves three constants which implies that at least three conditions are required to determine a circle uniquely.

11.1.3 Parabola
A parabola is the set of points P whose distances from a fixed point F in the plane are equal to their distances from a fixed line l in the plane. The fixed point F is called focus and the fixed line l the directrix of the parabola.

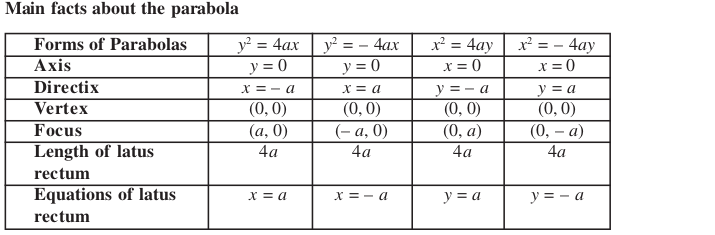
Standard equations of parabola
The four possible forms of parabola are shown below in Fig. 11.7 (a) to (d) The latus rectum of a parabola is a line segment perpendicular to the axis of the parabola, through the focus and whose end points lie on the parabola (Fig. 11.7).

Focal distance of a point
Let the equation of the parabola be y²= 4ax and P(x, y) be a point on it. Then the distance of P from the focus (a, 0) is called the focal distance of the point, i.e.,
1.1.4 Ellipse
An ellipse is the set of points in a plane, the sum of whose distances from two fixed points is constant. Alternatively, an ellipse is the set of all points in the plane whose distances from a fixed point in the plane bears a constant ratio, less than, to their distance from a fixed line in the plane. The fixed point is called focus, the fixed line a directrix and the constant ratio (e) the centricity of the ellipse.

We have two standard forms of the ellipse, i.e.,

Sum of the focal distances of any point on an ellipse is constant and equal to the length
of the major axis.

11.1.5 Hyperbola
A hyperbola is the set of all points in a plane, the difference of whose distances from two fixed points is constant. Alternatively, a hyperbola is the set of all points in a plane whose distances from a fixed point in the plane bears a constant ratio, greater than 1, to their distances from a fixed line in the plane. The fixed point is called a focus, the fixed line a directrix and the constant ratio denoted by e, the ecentricity
of the hyperbola.
We have two standard forms of the hyperbola, i.e.,