Candidates can download NCERT Exemplar Class 7 Maths Unit 8 from this page. The exemplar has been provided by the National Council of Educational Research & Training (NCERT) and the candidates can check it from below for free of cost. It contains objective, very short answer type, short answer type, and long answer type questions. Along with it, the answer for each question has also been provided. From the NCERT Exemplar Class 7 Maths Unit 8, candidates can understand the level and type of questions that are asked in the exam.
NCERT Exemplar Class 7 Maths Unit 8 Rational Numbers – PDF Download
| Document | Download |
|---|---|
| NCERT Exemplar Class 07 Maths Unit 8 Rationals Numbers | View PDF |
Read Online
NCERT Exemplar Class 7 Maths Unit 8 Rational Numbers
NCERT Class 7 Maths Unit 8 is for Rational Numbers. The type of questions that will be asked from NCERT Class 7 Maths Unit 8 are displayed in the below provided NCERT Exemplar Class 7 Maths Unit 8. With the help of it, candidates can prepare well for the examination.
Also Check: NCERT Solutions Class 7 Maths
Main Concepts and Results
A number that can be expressed in the form where p and q are integers and is called a rational number.
All integers and fractions are rational numbers.
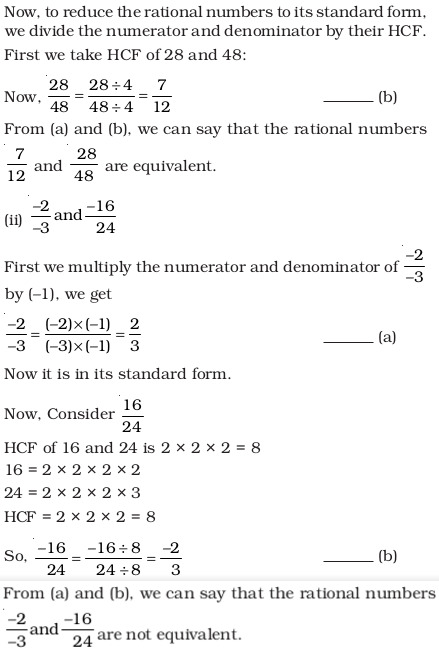
If the numerator and denominator of a rational number are multiplied or divided by a non-zero integer, we get a rational number which is said to be equivalent to the given rational number.
Rational numbers are classified as positive, zero or negative rational numbers. When the numerator and denominator both are positive integers or both are negative integers, it is a positive rational number.
When either the numerator or the denominator is a negative integer, it is a negative rational number.
The number 0 is neither a positive nor a negative rational number.
There are unlimited number of rational numbers between two rational numbers.
A rational number is said to be in the standard form, if its denominator is a positive integer and the numerator and denominator have no common factor other than 1.
Two rational numbers with the same denominator can be added by adding their numerators, keeping with the same denominator.
Two rational numbers with different denominators are added by first taking the LCM of the two denominators and then converting both the rational numbers to their equivalent forms having the LCM as the denominator and adding them as above.
While subtracting two rational numbers, we add the additive inverse of the rational number to be subtracted to the other rational number.
Product of rational numbers = .
The reciprocal of a non-zero rational number
To divide one rational number by the other non-zero rational number, we multiply the first rational number by the reciprocal of the other.
Solved Examples
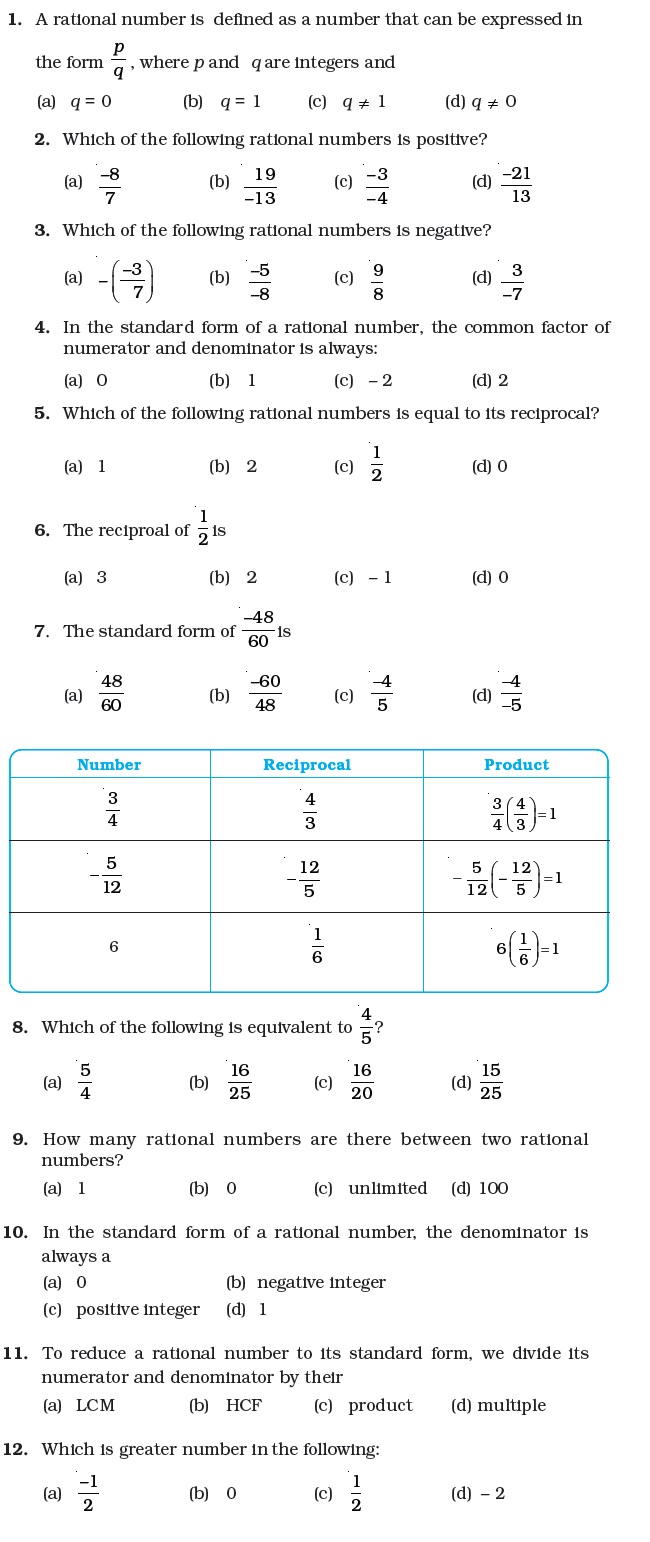
Multiple Choice Questions
Fill in the Blanks Type Questions
True False Type Questions
Other Important Type Questions
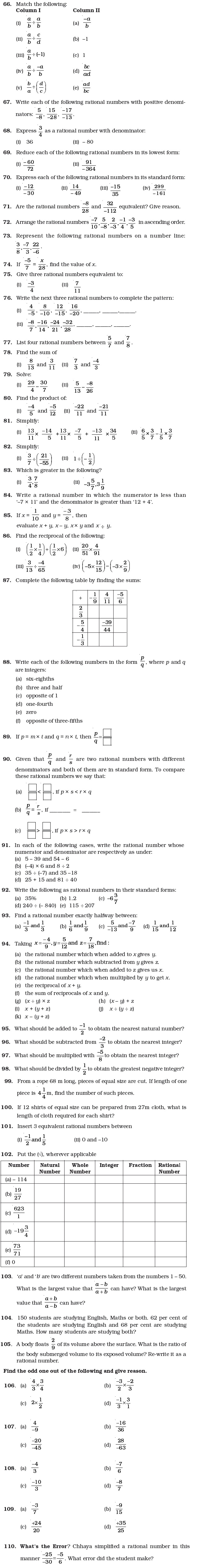
Application
Answers
NCERT Exemplar Class 7 Maths Unit 8 Rational Numbers – FAQs
How can I download NCERT Exemplar Class 7 Maths Unit 8 Rational Numbers?
Use the download table on this page – the PDF is free.
Can I read it online?
Yes – open the document to read it in your browser, or download it to read offline.