Candidates can download NCERT Exemplar Class 12 Maths Unit 2 from this page. The exemplar has been provided by the National Council of Educational Research & Training (NCERT) and the candidates can check it from below for free of cost. It contains objective, very short answer type, short answer type, and long answer type questions. Along with it, the answer for each question has also been provided. From the NCERT Exemplar Class 12 Maths Unit 2, candidates can understand the level and type of questions that are asked in the exam.
NCERT Exemplar Class 12 Maths Unit 2 Inverse Trigonometric Function
NCERT Class 12 Maths Unit 2 is for Inverse Trigonometric Function. The type of questions that will be asked from NCERT Class 12 Maths Unit 2 are displayed in the below provided NCERT Exemplar Class 12 Maths Unit 2. With the help of it, candidates can prepare well for the examination.
Also Check: NCERT Solutions for Class 12 Maths
2.1 Overview
2.1.1 Inverse function
Inverse of a function ‘f ’ exists, if the function is one-one and onto, i.e, bijective. Since trigonometric functions are many-one over their domains, we restrict their domains and co-domains in order to make them one-one and onto and then find their inverse. The domains and ranges (principal value branches) of inverse trigonometric functions are given below:

Notes:
(i) The symbol sin-1x should not be confused with (sinx)-1 . Infact sin-1x is an angle, the value of whose sine is x, similarly for other trigonometric functions.
(ii) The smallest numerical value, either positive or negative, of θ is called the principal value of the function.
(iii) Whenever no branch of an inverse trigonometric function is mentioned, we mean the principal value branch. The value of the inverse trigonometic function which lies in the range of principal branch is its principal value.
2.1.2 Graph of an inverse trigonometric function
The graph of an inverse trigonometric function can be obtained from the graph of original function by interchanging x-axis and y-axis, i.e, if (a, b) is a point on the graph of trigonometric function, then (b, a) becomes the corresponding point on the graph of its inverse trigonometric function.
It can be shown that the graph of an inverse function can be obtained from the corresponding graph of original function as a mirror image (i.e., reflection) along the line y = x.
2.1.3 Properties of inverse trigonometric functions


Short Answer Type Questions (Solved Examples)
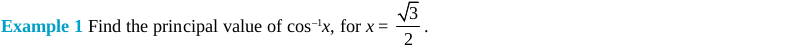






Long Answer Type Questions (Solved Examples)









Objective type questions (Solved Examples)



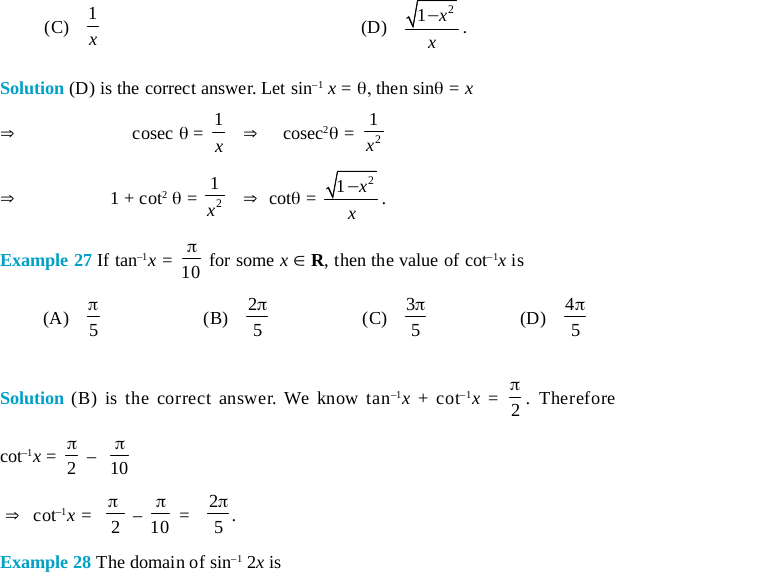

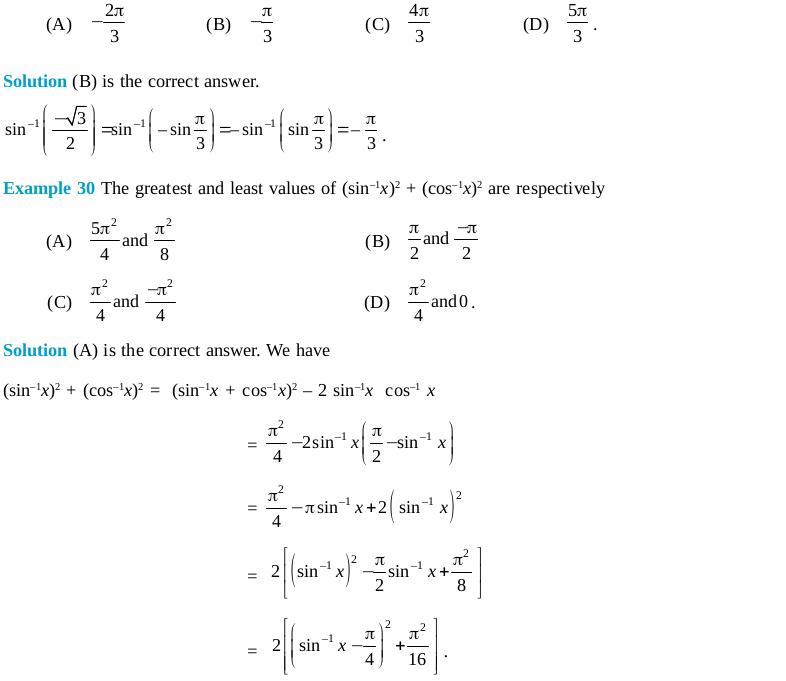

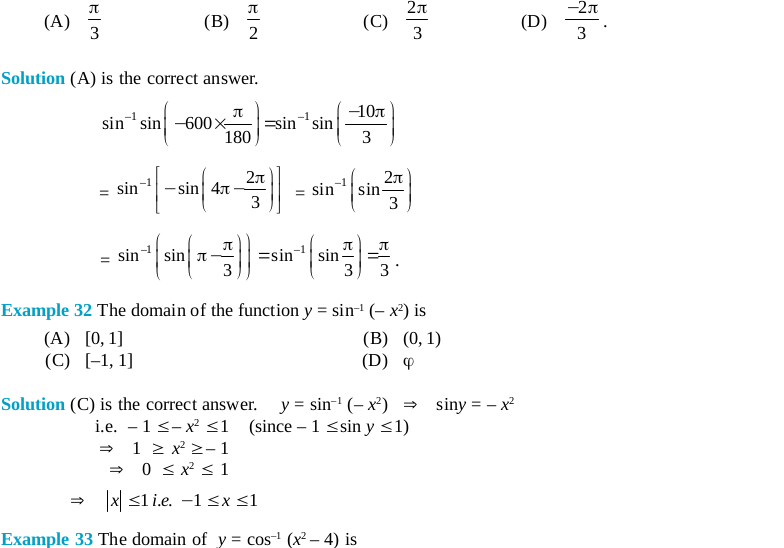






Short Answer Type Questions (Exercise)

Long Answer Type Questions (Exercise)
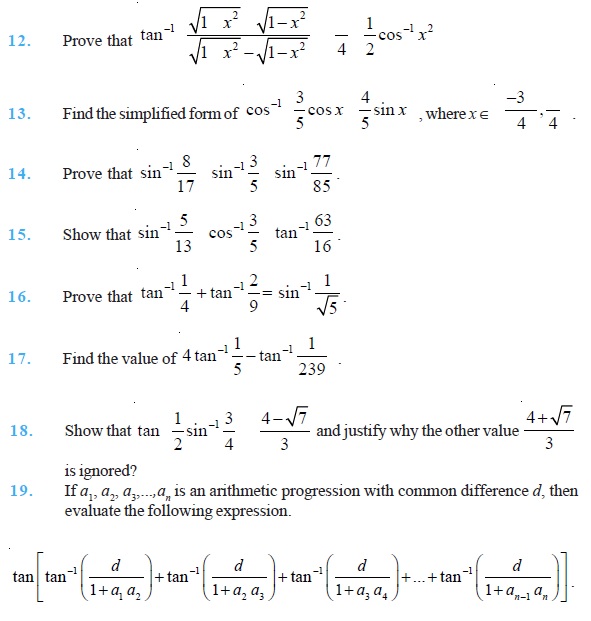
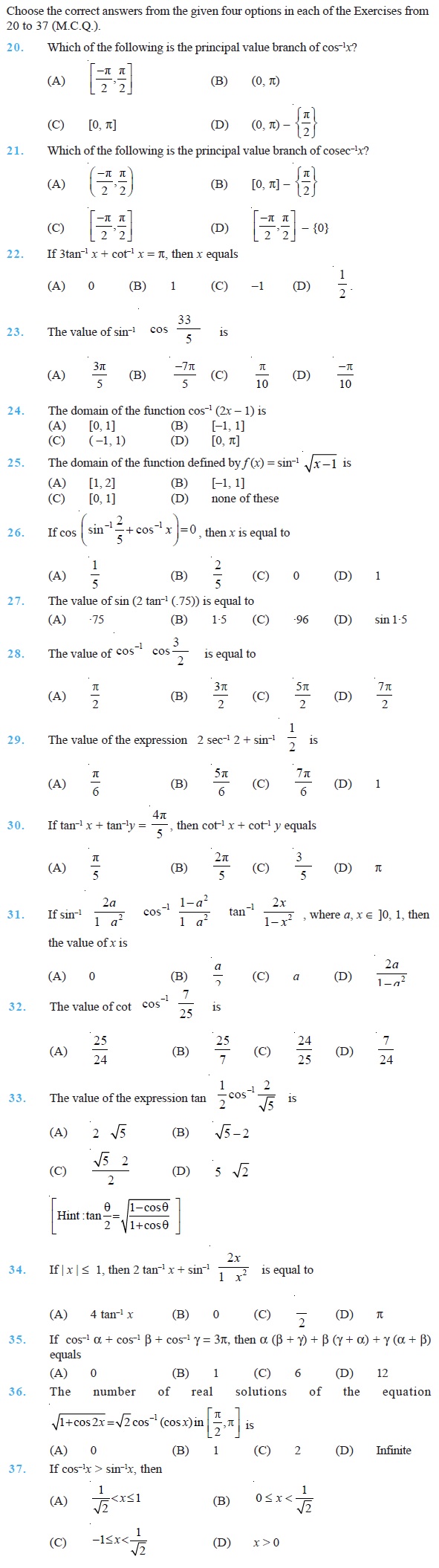
Multiple Choice Questions (Exercise)
Fill In Blanks Type Questions (Exercise)
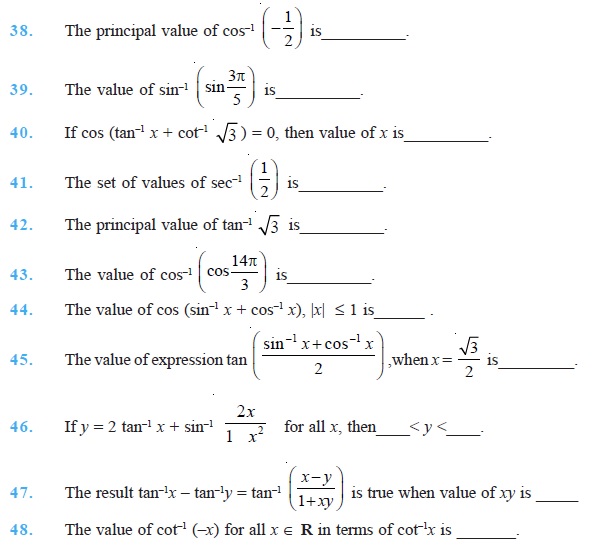
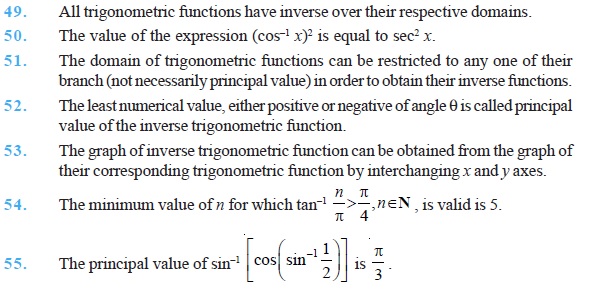
True or False Statements Type Questions (Exercise)
Click Here to download NCERT Exemplar Class 12 Maths Unit 2 Inverse Trigonometric Function.
Answers


Maths Physics Chemistry Biology
To get study material, exam alerts and news, join our Whatsapp Channel.

