Candidates can download NCERT Exemplar Class 12 Maths Unit 4 from this page. The exemplar has been provided by the National Council of Educational Research & Training (NCERT) and the candidates can check it from below for free of cost. It contains objective, very short answer type, short answer type, and long answer type questions. Along with it, the answer for each question has also been provided. From the NCERT Exemplar Class 12 Maths Unit 1, candidates can understand the level and type of questions that are asked in the exam.
NCERT Exemplar Class 12 Maths Unit 4 Determinants
NCERT Class 12 Maths Unit 4 is for Determinants. The type of questions that will be asked from NCERT Class 12 Maths Unit 4 are displayed in the below provided NCERT Exemplar Class 12 Maths Unit 4. With the help of it, candidates can prepare well for the examination.
Also Check: NCERT Solutions for Class 12 Maths
4.1 Overview
To every square matrix A = [aij] of order n, we can associate a number (real or complex) called determinant of the matrix A, written as det A, where aij is the (i,j)th element of A.

If A = , then determinant of A, denoted by |A| (or det A), is given by

|A| == ad – bc.
Remarks
(i) Only square matrices have determinants.
(ii) For a matrix A, |A| is read as determinant of A and not, as modulus of A.
4.1.1 Determinant of a matrix of order one
Let A = [a] be the matrix of order 1, then determinant of A is defined to be equal to a.
4.1.2 Determinant of a matrix of order two

Let A = [aij] = be a matrix of order 2. Then the determinant of A is defined as: det (A) = |A| = ad – bc.
4.1.3 Determinant of a matrix of order three
The determinant of a matrix of order three can be determined by expressing it in terms of second order determinants which is known as expansion of a determinant along a row (or a column). There are six ways of expanding a determinant of order 3 corresponding to each of three rows (R1 , R2 and R3 ) and three columns (C1 , C2 and C3 ) and each way gives the same value.
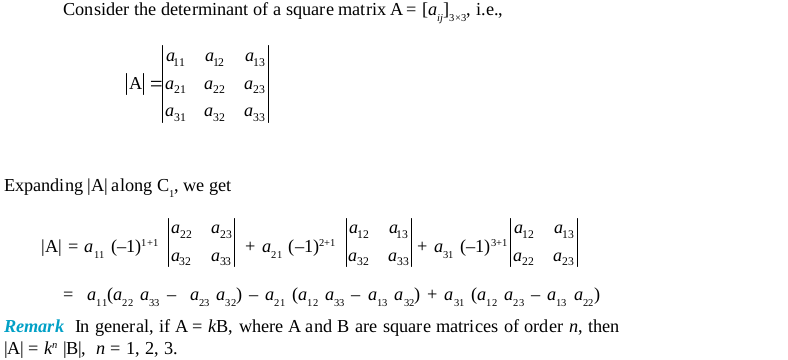
4.1.4 Properties of Determinants
(i) |A′| = |A|, where A′ = transpose of matrix A.
(ii) If we interchange any two rows (or columns), then sign of the determinant changes.
(iii) If any two rows or any two columns in a determinant are identical (or proportional), then the value of the determinant is zero.
(iv) Multiplying a determinant by k means multiplying the elements of only one row (or one column) by k.
(v) If we multiply each element of a row (or a column) of a determinant by constant k, then value of the determinant is multiplied by k.
(vi) If elements of a row (or a column) in a determinant can be expressed as the sum of two or more elements, then the given determinant can be expressed as the sum of two or more determinants.
(vii) If to each element of a row (or a column) of a determinant the equimultiples of corresponding elements of other rows (columns) are added, then value of determinant remains same.
Notes:
(i) If all the elements of a row (or column) are zeros, then the value of the determinant is zero.
(ii) If value of determinant ‘∆’ becomes zero by substituting x = α, then x – α is a factor of ‘∆’.
(iii) If all the elements of a determinant above or below the main diagonal consists of zeros, then the value of the determinant is equal to the product of diagonal elements.
4.1.5 Area of a triangle

4.1.6 Minors and co-factors

4.1.7 Adjoint and inverse of a matrix
(i) The adjoint of a square matrix A = [aij]n*n is defined as the transpose of the matrix.

(ii) A (adj A) = (adj A) A = |A| I, where A is square matrix of order n.
(iii) A square matrix A is said to be singular or non-singular according as |A| = 0 or |A| ≠ 0, respectively.
(iv) If A is a square matrix of order n, then |adj A| = |A|n-1 .
(v) If A and B are non-singular matrices of the same order, then AB and BA are also nonsingular matrices of the same order.
(vi) The determinant of the product of matrices is equal to product of their respective determinants, that is, |AB| = |A| |B|.
(vii) If AB = BA = I, where A and B are square matrices, then B is called inverse of A and is written as B = A-1. Also B-1 = (A-1)-1 = A.
(viii) A square matrix A is invertible if and only if A is non-singular matrix.
(ix) If A is an invertible matrix, then A-1 = (1/|A|) (adj A).
4.1.8 System of linear equations


Short Answer Type Questions (Solved Examples)



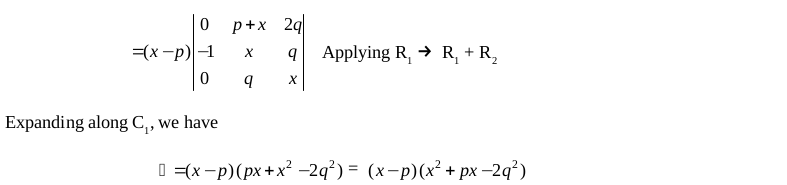

Long Answer Type Questions (Solved Examples)






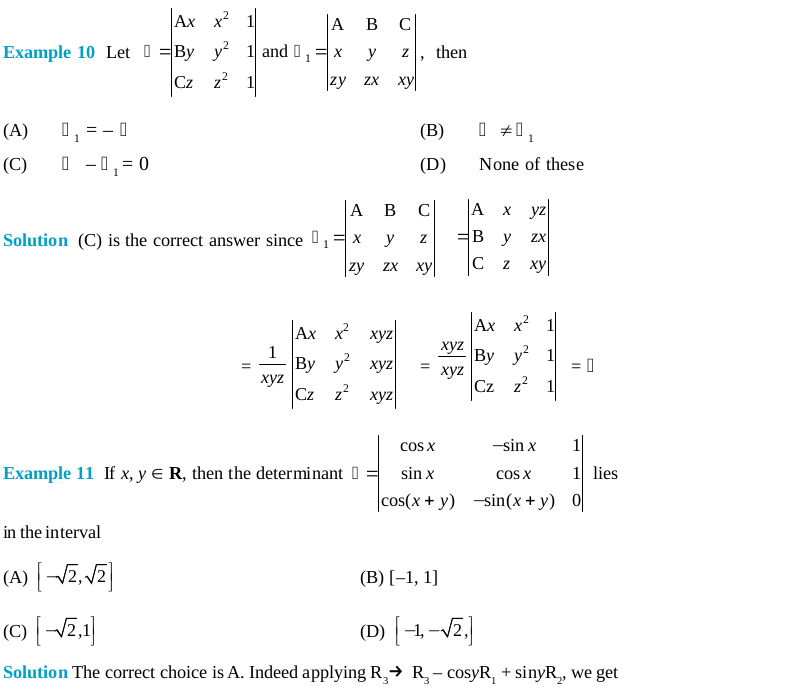
Multiple Choice Questions (Solved Examples)

Fill in the Blanks Type Questions (Solved Examples)


True or False Statements Type Questions (Solved Examples)


Short Answer Type Questions (Exercise)

Long Answer Type Questions (Exercise)
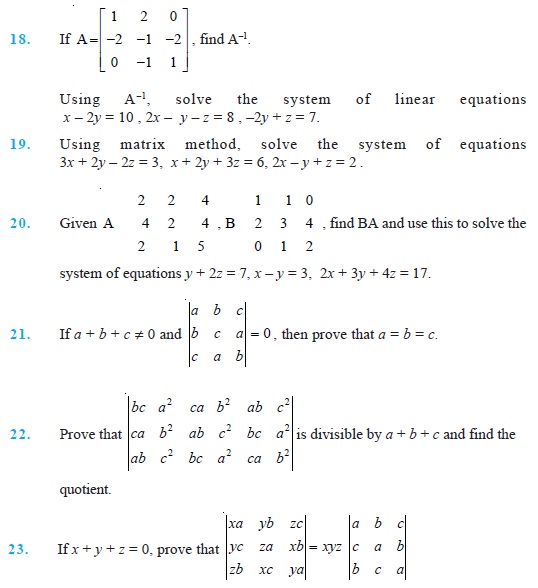
Multiple Choice Questions (Exercise)

Fill In Blanks Type Questions (Exercise)
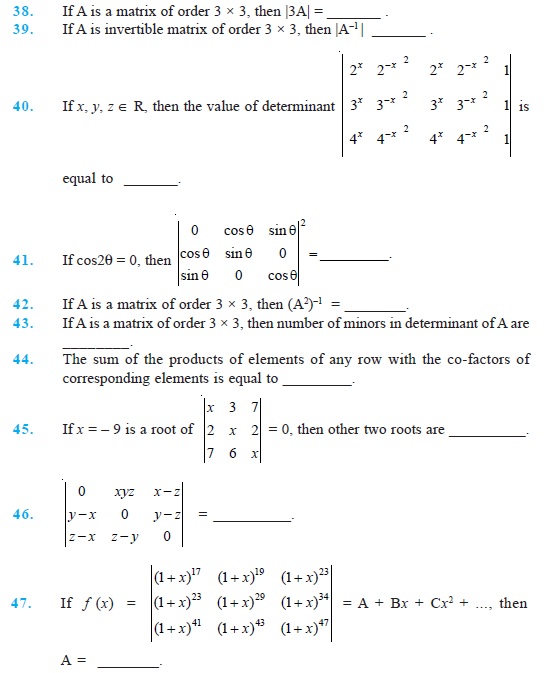
True or False Statements Type Questions (Exercise)
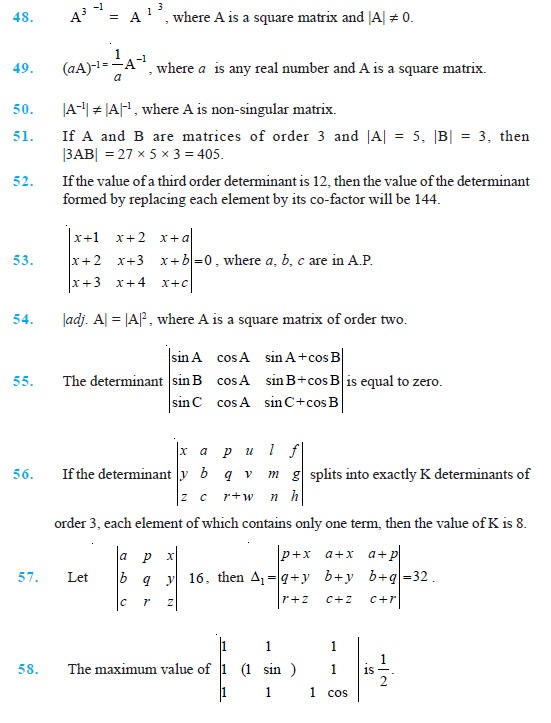
Click Here to download NCERT Exemplar Class 12 Maths Unit 4 Determinants.
Answers

Maths Physics Chemistry Biology
To get study material, exam alerts and news, join our Whatsapp Channel.
