Candidates can download NCERT Exemplar Class 12 Maths Unit 9 from this page. The exemplar has been provided by the National Council of Educational Research & Training (NCERT) and the candidates can check it from below for free of cost. It contains objective, very short answer type, short answer type, and long answer type questions. Along with it, the answer for each question has also been provided. From the NCERT Exemplar Class 12 Maths Unit 9, candidates can understand the level and type of questions that are asked in the exam.
Click Here for Class 12 Maths Notes
NCERT Exemplar Class 12 Maths Unit 9 Differential Equations
NCERT Class 12 Maths Unit 9 is for Differential Equations. The type of questions that will be asked from NCERT Class 12 Maths Unit 9 are displayed in the below provided NCERT Exemplar Class 12 Maths Unit 9. With the help of it, candidates can prepare well for the examination.
Also Check: NCERT Solutions for Class 12 Maths
9.1 Overview
(i) An equation involving derivative (derivatives) of the dependent variable with respect to independent variable (variables) is called a differential equation.
(ii) A differential equation involving derivatives of the dependent variable with respect to only one independent variable is called an ordinary differential equation and a differential equation involving derivatives with respect to more than one independent variables is called a partial differential equation.
(iii) Order of a differential equation is the order of the highest order derivative occurring in the differential equation.
(iv) Degree of a differential equation is defined if it is a polynomial equation in its derivatives.
(v) Degree (when defined) of a differential equation is the highest power (positive integer only) of the highest order derivative in it.
(vi) A relation between involved variables, which satisfy the given differential equation is called its solution. The solution which contains as many arbitrary constants as the order of the differential equation is called the general solution and the solution free from arbitrary constants is called particular solution.
(vii) To form a differential equation from a given function, we differentiate the function successively as many times as the number of arbitrary constants in the given function and then eliminate the arbitrary constants.
(viii) The order of a differential equation representing a family of curves is same as the number of arbitrary constants present in the equation corresponding to the family of curves.
(ix) ‘Variable separable method’ is used to solve such an equation in which variables can be separated completely, i.e., terms containing x should remain with dx and terms containing y should remain with dy.
(x) A function F (x, y) is said to be a homogeneous function of degree n if F (λx, λy )= λn F (x, y) for some non-zero constant λ.
(xi) A differential equation which can be expressed in the form dy/dx = F (x, y) or dx/dy = G (x, y), where F (x, y) and G (x, y) are homogeneous functions of degree zero, is called a homogeneous differential equation.
(xii) To solve a homogeneous differential equation of the type dy/dx = F (x, y), we make substitution y = vx and to solve a homogeneous differential equation of the type dx/dy = G (x, y), we make substitution x = vy.
(xiii) A differential equation of the form dy/dx + Py = Q, where P and Q are constants or functions of x only is known as a first order linear differential equation. Solution of such a differential equation is given by  where I.F. (Integrating Factor) =
where I.F. (Integrating Factor) =  .
.
(xiv) Another form of first order linear differential equation is dx/dy + P1x = Q1, where P1 and Q1 are constants or functions of y only. Solution of such a differential equation is given by

Short Answer Type Questions (Solved Examples)




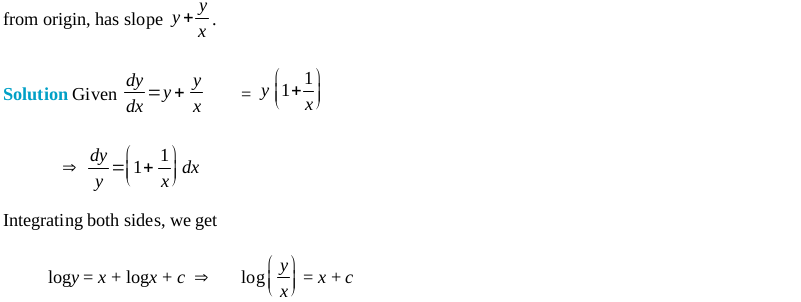

Long Answer Type Questions (Solved Examples)







Objective Type Questions (Solved Examples)





Fill in the Blanks (Solved Examples)




True or False Statements Type Questions (Solved Examples)




Short Answer Type Questions (Exercise)

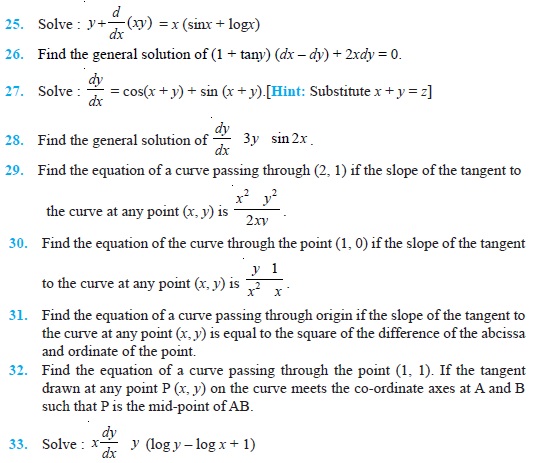
Long Answer Type Questions
Multiple Choice Questions

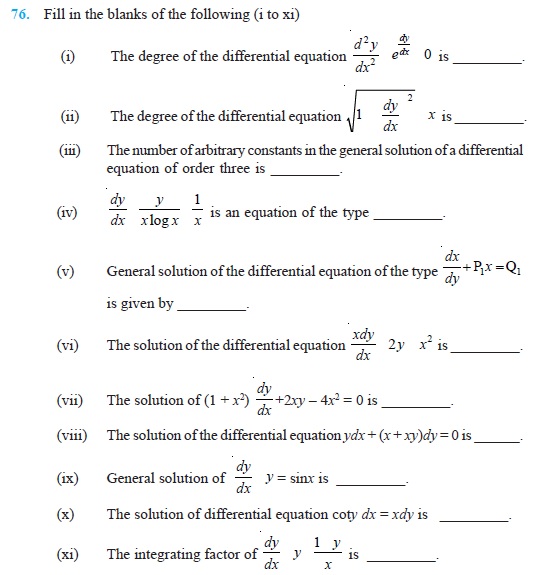
Fill In Blanks Type Questions
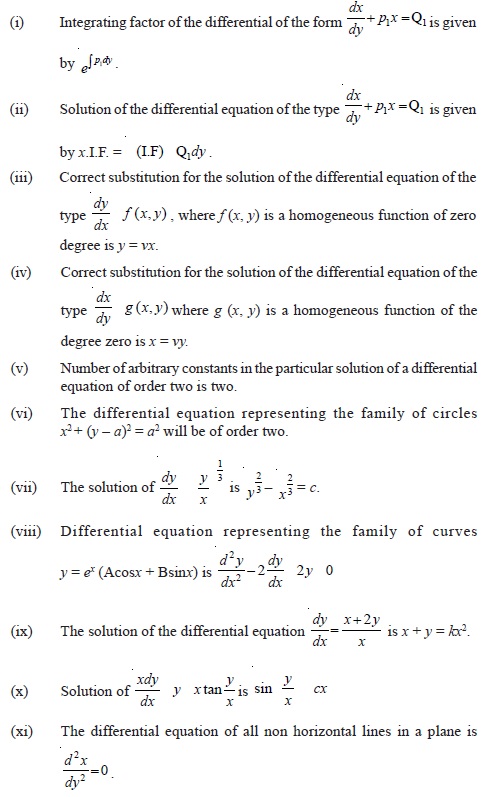
True or False Statements Type Questions
| « Previous | Next » |
Answers



Maths Physics Chemistry Biology
To get study material, exam alerts and news, join our Whatsapp Channel.


